is the heat transfer coefficient;
is the distance from the leading edge;
;
Jingquan Cheng
email: jcheng@nrao.edu
National Radio Astronomy Observatory
949 North Cherry Avenue
Tucson, AZ 85721
This memo discusses the efficiency change of the forced air cooling process when the site is located at 5,000 m above sea level. Formulae provided give theoretical numbers on efficiency loss. The calculation shows that for a larger characteristic length object, the loss in efficiency may be very large (78 %) since there is a transition between laminar to turbulent flow. For objects with smaller characteristic length, the efficiency loss will be of the order between 17 % and 39 %. At the same time, the memo also discusses the practical situation of the fan efficiency problem. The memo includes a number of useful figures of the cooling efficiency and a table of efficiency loss for electrical equipment at altitude.
In this discussion, we will use the following definitions:
is the heat transfer coefficient;
is the distance from the leading edge;
;
Table 1 lists the air properties which are of interest in the convection heat transfer process at sea level when the temperature is 0
C (Kreith 1986).
| Property | Magnitude | ||
| Density | | 1.252 |  |
| Specific heat | | 1011 | J/kg K |
| Thermal diffusivity | | 19.2 x |  |
| Thermal conductivity | | 0.0237 | W/m K |
| Absolute viscosity | | 17.456 x |  |
| Kinematic viscosity | | 13.942 x | |
| Prandtl number | Pr | 0.71 |
When the altitude changes, the air density changes rapidly as in Figure 1. For an altitude of 5000 m, the air density is 0.660 : 54 % that at sea level. Other parameters of interest are absolute viscosity and thermal conductivity. Hoerner (1965) states the absolute viscosity, , is a function of temperature and for most practical purposes is independent of pressure. At 0 C and at sea level, the absolute viscosity of air is 17.456x
. However, projection from data of Perry suggests a 2% drop when at an altitude of 5000 m due to pressure drop (Table 3-280 of Perry 1973). The thermal conductivty is even less affected by the altitude change. The change would be less then 0.2% (Table 3-288 of Perry 1973). Table 2 lists the air property data for an altitude of 5000 m. The change of kinematic viscosity is caused by the change of air density.
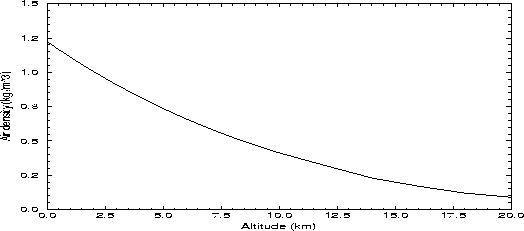
Figure 1: Air density as a function of altitude.
| Properties | Magnitude | Notes | ||
| Density | | 0.660 | | Ref: Gray |
| Thermal conductivity | | 0.0237 | W/m K | |
| Absolute viscosity | | 17.107 x | | Reduction of 2% |
| Kinematic viscosity | | 25.920x | | |
| Prandtl number | Pr | 0.71 |
Forced air cooling is a convection heat transfer process. The heat transferred is:
In representing the convectional heat transfer, the following dimensionless numbers are important. These are the Reynolds number, Prandtl number, and Nusselt number :
Among these quantities, Nu is directly related to the heat transfer coefficient, h. From the local value of Nu, the local value of can be obtained, and then an average heat transfer coefficient 
and an average 
number. For this reason, in the following we will use Nu or number to represent the heat transfer coefficient, h. Nu for a flow immersed body is also predicted from Re and Pr numbers by:
where and m are constants. The local convection coefficient is:
The average heat transfer coefficient is:
The average Nu number is:
Forced convection is complex due to the flow properties and shape of the object bodies. In this paper we only discuss two cases: plate case with its surface parallel to the air flow direction and cylinder case with its axis perpendicular to the flow direction. Other shaped bodies are not discussed in this memo, but similar effects would be expected. Laminar and turbulent flows are discussed. For a flat plate, when the Re number is less than 5 x 
, the plate cooling is under the laminar boundary-layer flow. There is no mixing of warmer and colder air particles by eddy motion and the heat transfer takes place solely by conduction. The coefficients in formula (5) are = 0.332 and m=0.5.
Using the same definition of average heat transfer coefficient, the average Nusselt number at this point is (p217 of Kreith 1986):
When the Re number is larger than 5 x , the cooling is a combination of laminar and turbulent flow. In reality, a laminar layer precedes the turbulent layer when the Re number is larger than 5 x . The transition from laminar flow to turbulent flow happens at a critical distance from the plate leading edge. In the transitional flow a certain amount of mixing occurs by means of eddies, which carry warmer air into colder regions, and vice versa. The mixing motion, even if it is only on a small scale, accelerates the transfer of heat considerably. Adding both the turbulent and laminar effects, the average Nusselt number for cases which include both laminar and turbulent flows is ( p232 of Kreith 1986):
The above mentioned case is rare in forced air cooling as vibration of fans or cooling objects can induce the transition from laminar to turbulent flow so near to the leading edge that, for practical purposes, the boundary layer is turbulent over the entire surface. At this time, there is no effect of laminar layer cooling. The average Nusselt number in this case when the Re number is between 5 x and 5 x 
will take the form of (p232 of Kreith 1986 and p476 of Janna 1986):
For other shaped bodies, e.g., circular cylinders axes perpendicular to the air flow, the general formula is the same as Equation (5). The coefficients are listed in Table 3 for laminar air cooling(Table 10-5 of Perry). The transition for cylinder shape cooling happens when the Re number is larger than 2.5 x .
| Re number | Pr number | | m |
| 1-4 | >0.6 | 0.989 | 0.330 |
| 4-40 | >0.6 | 0.911 | 0.385 |
| 40-4000 | >0.6 | 0.683 | 0.466 |
4x10 -4x10 | >0.6 | 0.193 | 0.618 |
4x10-2.5x10 | >0.6 | 0.0266 | 0.805 |
If the flow is within a pipe or a duct, the characteristic length is:
For a circular long pipe, the characteristic length is its diameter. The transition happens at Re number being 2000 to 5000 (p290 of Kreith). Before the transition, the Nu number is a function of 
and after the transition, the Nu number is a function of 
.
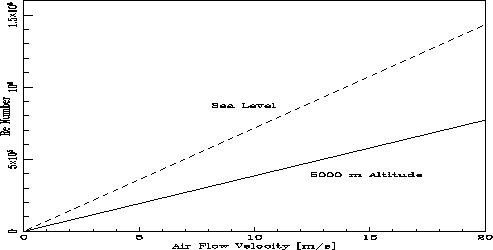
Figure 2: Air flow velocity and Re number at sea level and 5000 m altitude for 1 m long plate.
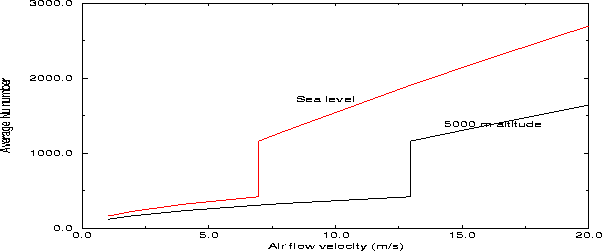
Figure 3: Nu number as a function of air flow velocity at sea level and 5000 m altitude for 1 m long plate.
The characteristic length, L, of a plate parallel to the flow is its length. From the formulae and data provided in the previous sections, we could calculate the Re and Nu number change when the site is moved to a 5000 m altitude. Figure 2 here is the Re number for different air flow velocity when the characteristic length is equal to 1 m. From this figure, the change in altitude will change the Re number significantly. This is important as the transition between the laminar and the turbulent layers for a plate parallel to the flow will happen at Re = 5 x . Using the Re number calculated in the average Nu number formulae, the results are shown in Figure 3. The abrupt transitions in the figure are caused by the transition between laminar flow and turbulent flow. The cooling efficiency will drop greatly if the velocity is between these two vertical lines. For a plate length much greater than 1 m, the transition will be in a even lower velocity range. In this figure, we assume that no laminar layers precede the turbulent layers on the plate surface. This is true for most forced air cooling processes. The efficiency loss is:
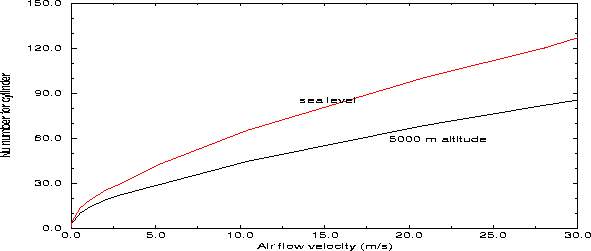
Figure 4: Nu number as a function of air flow velocity at sea level and 5000 m altitude for 0.02 m diameter cylinder.
The characteristic length of a cylinder perpendicular to the flow is its diameter. For a characteristic length of 0.02 m, the Re number is reduced by 50 times that for a characteristic length of 1 m,. The flow is always within laminar range ( Re 
2.5 x for cylinders). However, the coefficient for cylinder calculations is related to the Re number as shown in Table 3. A higher Re number will result in a higher power in the Nu number calculation. Figure 4 shows the curves of Nu as a function of flow velocity. The general trend in the figure is that the lower the flow velocity, the smaller the efficiency loss. The exact efficiency loss in this figure is:
For electronic cabin cooling, since the dimensions of the components being cooled are limited to 0.05 - 0.1 m, the Re number in most cases is always small and the cooling process is within the laminar range. The efficiency loss at high altitude could be simply represented by 
(range from 17% to 39%), where m ranges from 0.3 to 0.8 depending upon the shape of the components and the Re number.
For a fixed fan, the air flow volume is constant. Figure 5 is provided here from a catalog of a fan manufacturer ( Comair Rotron, 1992). From the figure, the fan performance is greatly reduced if the fan is used at increased altitude. Usually, the air flow velocity is fixed for a particular fan. When the air density is reduced, the Re number will be reduced in the same ratio as the density. The Re number determines the Nu number which is an indicator of the cooling efficiency. In cases where the fan speed changes, the basic fan laws state that the air flow volume varies directly with the fan speed ratio, the air pressure varies with square at speed ratio, and the power varies with cube of speed ratio. The horsepower also varies with the air density. By increasing the fan speed 22 % at a 5000 m altitude (the air density is 54 % of that at sea level), the horsepower required from the fan motor remains constant. However, the Re number will be reduced by a factor of 34 %. The Nu number would also be reduced. This assumes that the fan motor does not have efficiency loss.
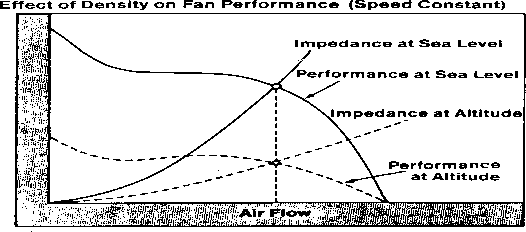
Figure 5: Fan performance change as the site altitude changes.
| Item | 2000 m | 3000 m | 4000 m | 5000 m |
| Diesel engines | 25% | 40% | 55% | 70% |
| Air compressors | 35% | 55% | 75% | 95% |
| Vacuum pumps | 20% | 30% | 40% | 50% |
| Transmission lines | 10% | 20% | 30% | 40% |
| Transformers | 5% | 15% | 25% | 35% |
| Electrical machines | 5% | 15% | 25% | 35% |
Air density change also affects the cooling of electrical equipment. The electrical equipment would produce heat during operation. The heat is dissipated mostly via forced air cooling within the equipment. Generally, larger equipment will have greater loss in efficiency in forced air cooling as the characteristic length, L, is a determining factor in the calculation of the Re number. The Re number may reach a transition from laminar flow to turbulent flow when the altitude is low, while it may be below the transition when the altitude is high. For example, the diesel engine has dimensions near to or larger than 1 m. The reduction in cooling efficiency may be significant. Of course, thinner air at altitude is an addtional factor in reducing the engine efficiency. This is not discussed in this memo. In an article by Jimenez, there is a table for correction in output of electrical equipment when at altitude. The table does not have other descriptions and is included for reference (Table 4). However it agrees well in most cases with our prediction on the forced air cooling.
Forced air cooling at altitude will be seriously affected if the characteristic length of the object is about 1 m or larger (in a particular case, a reduction could be as large as 78 %). However a proportional reduction between 17% and 39% in efficiency is expected for all small size objects. For electrical equipment, Table 4 is a good estimation for the efficiency loss at altitude.
Jeff Hagen and Mark Gordon provided important information on heat transfer and Jennifer Neighbours helped in English.
Comair Rotron, 1992, Catalog of fans, blowers and accessories.
Gray, D. F., American Institute of Physics Handbook, McGraw-Hill Co..
Hoerner, S. F., 1965, Fluid-dymanic drag, Published by author.
Janna, W. S., 1986, Engineering heat transfer, PWS Pub., Boston.
Jimenez, D., Chapter 25: High altitude intermittent chronic exposure: Andean mines. Source unknown.
Kreith, F. & Bohn, M. S., 1986, Principles of heat transfer, 4th edition, Harper & Row, Pub, New York.
Perry, R. H. & Chilton, C. H., 1973, Chemical engineers' handbook, McGraw-hill Book co., New York.
